NOTE: This post was originally published at my other blog  April 15, 2017.

How much of the math you do in your classroom is based on someone else’s questions? Someone far away from your classroom? Someone other than your students? Even with specter of standardized tests looming like dark, heavy clouds, how can we leave room for the most important part of learning: question asking?

“So many of the things that we do in math education — and maybe more generally in education — are giving students answers to questions that they would never think of asking. By definition, that’s what it is to be boring. If you’re sitting at a bar and someone’s telling you stuff that you’re not interested in and you would never think of asking about — what is more boring than that? That seems to be the model of our educational system: ‘Here’s the formula for the cosine of the double angle.’ ‘Well, I don’t care about that.’” —Steven Strogatz

The first time I experimented with building scaled-up geometric forms I was homeschooling my then-seven-year-old. At the time she was a “resistant” learner which basically meant she was happiest exploring her own questions, and, over a couple years of homeschooling I realized my best strategy was to influence the child by way of my own curiosities and the way I structured the environment, leaving out provocations to be discovered and, if of interest, investigated.  This was also a time when I was deep into finding answers for my own question “What is math?” and I was heavy into an investigation into Platonic Solids. I had never been a builder as a child, but I had a question and it needed to be answered.

Back in April, on Twitter, I had a conversation with Lana, a third grade teacher who is reading Math on the Move and who has been wondering about how how to “scale up” her students’ mathematical activity.  Specifically she’s been curious about my recent work with building body-scale polyhedra.  Body- or moving-scale means that the whole body/person is engaged in problem solving and mathematical thinking to investigate a mathematical challenge or project of some kind. I think Telanna’s question could be anybody’s question who is wondering how we can do math off the page.

My answer focused on how I structure a making activity and the learning environment in a way that motivates learners to collaborate and ask new questions in response to the activity in an intrinsic way.

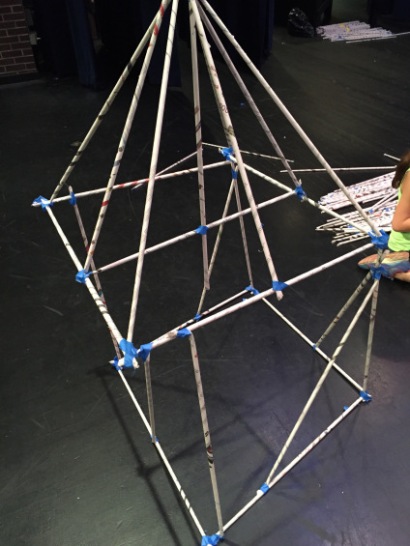
It’s in the process of making something with the freedom to try things out and see where it gets you that creates new questions.

It’s these questions, arising in the moments when they’re needed, born of collaboration,   that help learners notice structure and pattern and purpose in what they’re doing. From there we can move to the more formal learning. But, like my daughter, I think kids in general are most motivated when they are provided agency by the adults in their lives. Their work may not be technically perfect, but they are in the best part of learning (to me, anyhow): inside the flow of an investigation filled with their wonderings.

To bring kids to math we need to leave room for their own questions.

What happens next? There are more questions to be asked about this kind of approach. I have  my answers and am happy to share them. But I’d love to hear your questions first!

Malke Rosenfeld is a dance teaching artist, author, editor, math explorer, and presenter whose interests focus on the learning that happens at the intersection of math and the moving body. She delights in creating rich environments in which children and adults can explore, make, play, and talk math based on their own questions and inclinations.You can find out more about her work at malkerosenfeld.com,  on Twitter,  Instagram, or Facebook.